Work and energy in linear and angular dynamics

Dr. Shahid Ali Yousafzai

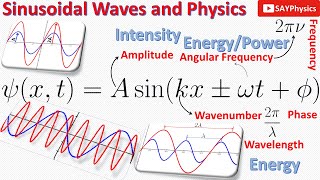
We will discuss the terminology of work. We can define the work in linear dynamics as well as in angular dynamics. In linear dynamics or in translational dynamics work is $$ W=\vec { F } .\vec { x } $$ $$ dW=\vec { F } .d\vec { x } $$ and if I integrate both sides then $$ \int { dW } =\int { \vec { F } .d\vec { x } } $$ $$ W=\int { \vec { F } .d\vec { x } } $$ In angular dynamics I can write that $$ W=\vec { \tau } .\vec { \theta } $$ $$ \int { dW } =\int { \vec { \tau } .d\vec { \theta } } $$ $$ W=\int { \vec { \tau } .d\vec { \theta } } $$ Now if I want to know that how quickly this work is done, which is actually power and is defined as $$P=\frac { W }{ t } =\frac { \vec { F } .\vec { x } }{ t } \vec { F } .\vec { v } $$ Similarly $$ P=\frac { W }{ t } =\frac { \vec { \tau } .\vec { \theta } }{ t } \vec { \tau } .\vec { \omega } $$ If I want to focus on the kinetic energy, so $$ T=\frac { 1 }{ 2 } m{ v }^{ 2 }$$ I know from my previous calculation that $$ T=\frac { 1 }{ 2 } m{ v }^{ 2 }=\frac { 1 }{ 2 } m{ r }^{ 2 }{ \omega }^{ 2 }=\frac { 1 }{ 2 } I{ \omega }^{ 2 } $$ where $I$ the is moment of inertia. The role played by mass in translational dynamics is played by moment of Inertia in rotational dynamics. This is the difference between terminologies for the work.