State and Explain the Coulombs Law

Coulomb performed a number of experiments to see the effect of placing two small charges near each other. On his experimental researches, he established a law which is known as Coulomb's Law of Electrostatics.

According to Coulomb's Law : The force of attraction or repulsion between two charges is directly proportional to the product of magnitude of the two charges and inversely proportional to the square of distance between them.

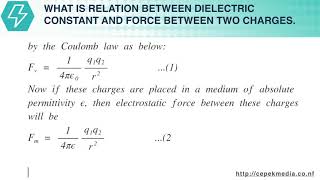
If two point charges ${ q }_{ 1 }$ and ${ q }_{ 2 }$ have distance $d$ between them, then force $F$ between the charges can be mathematically expressed as: $$F \propto { q }_{ 1 }{ q }_{ 2 }\qquad \qquad \qquad ...(1)$$ $$F \propto \frac { 1 }{ { d }^{ 2 } }\qquad \qquad \qquad ...(2)$$ Combining (1) and (2), $$F \propto \frac { { q }_{ 1 }{ q }_{ 2 } }{ { d }^{ 2 } }$$ or $F = K\frac { { q }_{ 1 }{ q }_{ 2 } }{ { d }^{ 2 } }\qquad \qquad \qquad ...(3)$

Where, $K$ is a constant of proportionality and its value depends upon the medium in which the charges are placed and the system of units used. In SI units force is measured in newton, charge in coulomb, distance in metre and the value of $K$ is given as, $$K = \frac { 1 }{ 4\pi { \epsilon }_{ 0 }{ \epsilon }_{ r } }\qquad \qquad \qquad ...(4)$$

where, ${ \epsilon }_{ 0 } =$ Absolute permittivity of vacuum or permittivity of free space $= 8.854 \times { 10 }^{ -12 }$ farad/metre

${ \epsilon }_{ r } =$ Relative permittivity of the medium w.r.t. vacuum in which the charges are placed (e.g. for air ${ \epsilon }_{ r } = 1$)

Putting the value of $K$ in equation (3), we get, $$\boxed { F = \left( \frac { 1 }{ 4\pi { \epsilon }_{ 0 }{ \epsilon }_{ r } } \right) \frac { { q }_{ 1 }{ q }_{ 2 } }{ { d }^{ 2 } } } \qquad ... (5)$$

Unit Charge or one coulomb charge in SI system can be defined as the amount of charge which when placed at a distance of one metre from an equal and similar charge in air, is repelled with a force of $9 \times { 10 }^{ 9 } newton$ from it.

As per this defination, $${ q }_{ 1 } = { q }_{ 2 } = q,$$ $$F = 9 \times { 10 }^{ 9 } newton,$$ $${ \epsilon }_{ 0 } = 8.854 \times { 10 }^{ -12 } farad/metre,$$ ${ \epsilon }_{ r } = 1$ (Assuming two charges to be placed in air) $$d = 1m$$

Putting these values in the above relation, we get $$9 \times {10 }^{ 9 } = \frac { q \times q }{ 4\pi \times \left( 8.854 \times { 10 }^{ -12 } \right) \times 1 \times { 1 }^{ 2 } }$$ $$9 \times { 10 }^{ 9 } = \frac { { q }^{ 2 } }{ 4\pi \times \left( 8.854 \times { 10 }^{ -12 } \right) }$$ $${ q }^{ 2 } = \left( 9 \times { 10 }^{ 9 } \right) \times 4\pi \times \left( 8.854 \times { 10 }^{ -12 } \right)$$ $$= 1.00086$$ $\therefore q = \pm 1.00043$ coulomb

Hence, unit charge.